The Fibonacci sequence is a series of numbers made famous by Leonardo Fibonacci in the 12th century. It has been described in texts for over two millennia, with the earliest description found in Indian texts in 200 BC, and further development throughout the first millennium. It appears commonly in mathematics and in nature, and for that reason has become a popular pedagogical tool.
Why Is the Fibonacci Sequence Important?
The Fibonacci sequence has many interesting properties and appears in various areas of mathematics, science and nature. Given how often it can be found in nature, some have suggested that the sequence has some underlying mathematical principles at work in nature. The Fibonacci Sequence also has connections to other areas of mathematics such as number theory, algebra and geometry.
How Does the Fibonacci Sequence Work?
The formula that defines the Fibonacci sequence is:
Fn=Fn-1+Fn-2
We can also describe this by stating that any number in the Fibonacci sequence is the sum of the previous two numbers.
For the most common representation of the Fibonacci sequence, the first two terms are defined as F0=0, F1=1. This leads to the sequence 0, 1, 1, 2, 3, 5, 8, 13, . . .
It’s possible to calculate other Fibonacci sequences by starting with different base numbers, for example:
F0=0, F1=2; Fibonacci sequence: 0, 2, 2, 4, 6, 10, 16, 26, . . .
F0=2, F1=1; Fibonacci sequence: 2, 1, 3, 4, 7, 11, 18, 29, 47, . . .
The Fibonacci sequence also has a closed form representation, known as Binet’s formula. With the closed formula it’s possible to calculate the nth value in the Fibonacci sequence directly, without calculating each of the previous numbers.
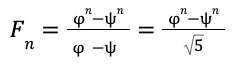
where
![]()
Is the Fibonacci Sequence the Same as the Golden Ratio?
No, the Fibonacci sequence and the golden ratio are not the same. That said, the Fibonacci sequence is intimately related to the golden ratio, a value with significant cultural importance. The golden ratio has fascinated people across numerous fields, from art to architecture to music.
The golden ratio can be approximately derived by dividing any Fibonacci number by the previous one. This ratio becomes more accurate the further you proceed down the sequence. You can see the results below.
1/1 = 1
2/1 = 2
3/2 = 1.5
5/3 = 1.3
. . .
89/55 = 1.62
. . .
Fn/Fn-1 ≈ 1.618 . . .
What Are Other Sequences Similar to the Fibonacci Sequence?
The Fibonacci sequence is an example of a number sequence, of which there are many others in mathematics. Some other common sequences include:
Arithmetic Sequence
You can reach each number by adding a fixed number to the previous one. Each pair of numbers has a common difference.
An = An-1+a1 = a0+ n x a11, 3, 5, 7, 9, 11, . . .
Triangular Numbers
The nth triangle number is the number of dots in a triangle with n dots on a side. You can also state it as the sum of all the numbers from 1 to n.
Tn = n(n+1) / 21, 3, 6, 10, 15, 21, . . .
Magic Squares Constant
In magic squares, a set of numbers is arranged in a square to such that the rows, columns and diagonals all sum up to the same value. A magic square of size n is typically filled with the numbers from 1 to n2. The common sum is known as the magic constant.
Mn=n(n2+1) / 21, 5, 15, 34, 65, 111, . . .
Fibonacci Sequence In Science
Fibonacci search is a key application of the Fibonacci sequence in the space of computer science. In Fibonacci search, the search space is divided up into segments according to the Fibonacci numbers, differing from common search algorithms such as binary search. This algorithm isn’t commonly used today, but it has niche applications.
For example, when the array you’re searching is very large and cannot fit in memory, Fibonacci search can be more efficient. You can also use Fibonacci search when only the addition and subtraction operations are available, as opposed to binary search which requires division or multiplication. However, on average, Fibonacci search requires four percent more comparisons compared to binary search.
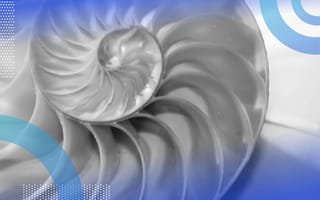
The Fibonacci Sequence in Nature and Art
Outside of human applications, we find the Fibonacci sequence in nature. For example, the arrangement of seeds on pinecones follow the Fibonacci sequence, as do the seeds in a sunflower and the sections of a Roman cauliflower.

These various applications are interesting discoveries, however there has been no strong justification for why these various phenomena occur in nature. Similarly, in various artworks and architectural findings, there is limited evidence that the creators specifically built the Fibonnaci sequence into their works.
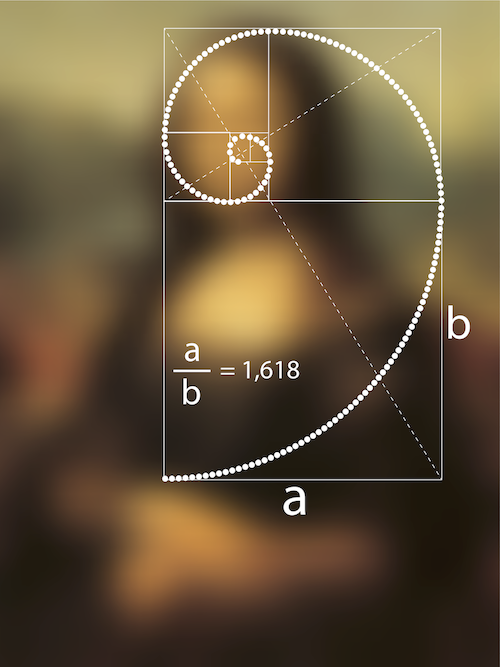
Humans are good at finding patterns, even when no patterns exist. In the case of Fibonacci, we have to be careful not to over-analyze unrelated patterns.